Introduction
It is an accepted fact that time passes more slowly for objects travelling near the speed of light. This shows that the rate at which time passes is a variable: it is not fixed. This article extends this fact to postulate that the passage of time as we experience it is not the same as it was when the universe originated, and furthermore, that the rate at which time passes in any given situation is related to the available energy.
As there was vastly more energy (and no matter) available at the origin of the universe than there has been at any time since, the clock rate would have been very high: the “big bang” took many more years than is currently accepted (because the “years” were much shorter then.)
It is furthermore suggested, that this energy had to come from some source, and a collapsing anti-matter universe would give symmetry to the situation. This is analogous to a resonating violin string where there is an oscillation between potential energy and kinetic energy, or an electrical resonance, where the oscillation is between electrostatic energy (voltage across a capacitor) and electromagnetic energy (current in an inductor).
In the case of the Universe, it is proposed that the resonance is between energy (and clock rate) on the one hand, and matter (mass and space) on the other, though not at the clock rate as we are currently experiencing.
The mathematics
The term Progression angle has been coined herein for the axis along which the resonance takes place. It is given an angular dimension solely to avoid confusion with a time axis. Similarly, any words with time connotations used as relating to this Progression angle instead of to time, are suffixed with a "~". eg. rate~, continuous~, repetition~, etc.
Initially, a simplified homogeneous universe is described in which all matter is in phase~ (ie. the mass, if any, is distributed evenly throughout the space), and where energy and clock rate~) are similarly in phase~. Later the possible effects of these variables being out of phase~ and the significance of the speed of light as a limit will also be considered.
Figure 1 shows generalised curves representing mass (m), space (v), energy (e) and clock rate (r) of the form
m = M sin ø (1)
v = Vsin ø (2).
e = E cos ø (3)
r = R cos ø (4)
(In the figure, the values of M, V, E & R are normalised to 1. The Progression angle (ø) is shown in radians.)
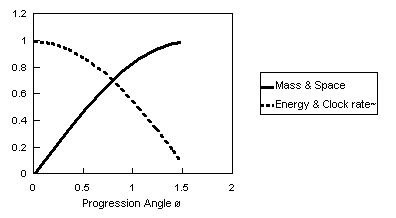 Fig.
1
Fig.
1
Note that in the universe as we experience it, the rate at which time passes has to run very fast to begin with, and slow to zero as the progression angle reaches π/2. This implies that time "t" as we measure it, is given by 1/r.
Plotting the curves of fig. 1 against time "t" as we know it, results in Fig. 2, and that looks remarkably like the big-bang. (The time units in fig. 2 are arbitrary.)
(At progression angle zero, the time scale in fig 2, only goes back to unity, and not zero. It is limited by the value of R which can not be infinite.)
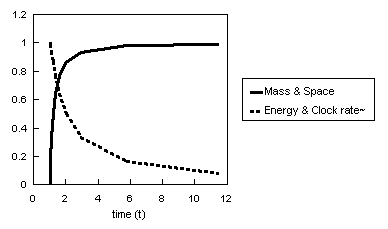 Fig.
2
Fig.
2
The other quadrants
Our universe, exists for progression angles from 0 to π/2 (Quadrant 1). The previous~ quadrant, (quadrant 4 from -π/2 to 0) contains a collapsing~ anti-matter universe where matter is turning into energy at an ever-increasing rate~.
The quadrant following~ ours (2) contains a collapsing~ matter universe, but as time is running backwards compared to us, it presumably behaves like our universe to any inhabitants. However, not only time is negative, so also is energy. Presumably this is anti-energy (analogous to anti-matter?)
The remaining quadrant (3) contains a universe with (anti-) energy turning~ into anti-matter, again with time running backwards, so it behaves like quadrant 4 to any inhabitants.
Phase~ differences.
Up to now, a homogeneous universe has been considered, but it is not, of course. The mass is concentrated into stars and planets. Gravity affects the clock. It is already known that light is bent when passing near to large planetary masses. This corresponds to a slowing down of the clock: the region exists at a later~ progression angle. As the universe as a whole nears a progression angle of π/2 one would expect to find progressively more matter entering this “final” (to us) stage. This describes black holes (where light can not escape because the clock rate has fallen to zero and where it can therefore “wait” for the rest of the universe to catch up.)
Speed of light.
In this theory, the universe we inhabit starts with a finite amount of energy, and ends with a finite amount of matter. There should therefore be some definable maximum limit. This has to be related to the speed of light (c), but speed is measured as distance/time, both of which are variable in this model. Taking the whole universe and applying the equations (1) and (4) given above Fig.1, gives:
c = m/t = MR sin ø. cos ø. (5)
This has a maximum value of ½ MR, but it falls to zero: at 0 (when there is no space )and π/2 (when the clock stops). Applying very small “c” near the beginning and end of the universe to the well known e=mc2 formula shows that almost no energy was needed at the beginning to produce the matter and almost no energy will be left to extract at the end.
It is also of note that because R and E are directly related, the maximum value of the speed of light is also determined by the product of M and E, though no attempt is being made to solve this equation.
The “missing” energy.
A final thought: there is alleged to be insufficient mass in the universe to account for all the energy. But space itself had also to be created. It is suggested that this additional energy was used to create the non-mass space.
Postscript.
It must be stressed that the author is not a physicist, and is not in a position to validate this work by any practical experimentation, but the work does appear to fit many of the known facts.
A.J. Tricker.